Effects of the ended length of the registries
1) binary Representation of numbers decimates them :
In short to every bit a power of base two must be associated, such power is positive beginning from 0 for the figures to right of the binary virgola while it is negative beginning from â?"1 for the figures on the left of the same one.
2) binary Arithmetic in fixed virgola :
The position of the binary virgola that separates the sequence that describes the entire part of the number from the sequence that describes the fractional part is fixed. They observe that the result of the multiplication operation exceeds the dimensions of the registry and is therefore necessary to rerun to the troncamento or to the rounding while the operation of sum from place to a saturation that only can be avoided using the representation in mobile virgola.
3) Representation in mobile virgola :
Every positive number is represented like F = 2c M where c is said characteristic that it can be is positive that negative while M are said mantissa and are a number comprised between ½ and 1.
The product is come true multiplying the mantisse as they were fractions to fixed virgola and adding the characteristics while the sum is carried out by means of an operation of translation, in both the cases it can exceed the dimensions of the registry and is therefore necessary to resort to the troncamento or to the rounding.
4) main Difference between the representation in fixed virgola and that one in mobile virgola:
The representation in mobile virgola necessity of rounding or troncamento is for the multiplication that for the sum while for the representation in fixed virgola that is necessary only for the multiplication, but the addition can give place to saturation.
5) Rappresentazioni of the numbers denied to you :
In order to represent numbers denied to you to fixed virgola the three methods are available following:
to) the module it is represented by means of a binary number whose sign is given from the binary figure of head.
In short the first bit of the representation is dedicated to the sign while remaining the bit characterizes the module of the fraction
b) Complement to one
The positive fractions are represented in module and sign while the negative fractions are representative complementando all the bit that is laddove is 1 replaces 0 and viceversa.
c) Complement to one
The positive fractions are represented in terms of module and sign while the negative fractions are obtained embezzling of the module from 2,0.
6) Amplitude of quantization :
It is the numerical value of a 1 in the less meaningful bit, it is worth 2- b .
7) Error of troncamento for the representation to fixed virgola:
where Q[x ] is the number after troncamento and x is the number before the troncamento and for positive numbers there is a negative amount while for numbers denied there is a positive amount if they are represent to you in complement to two while it is a negative amount if they are represents to you in complement to one or in module and sign.
8) Error of rounding for the representation to fixed virgola:
such error is independent from the way in which the numbers denied to you are represented.
9) relative Error in the case of the rounding in the representation to mobile virgola:
10) relative Error in the case of the troncamento in the representation to fixed virgola:
If the representation is in complement to or in module
and a sign it has 
while for the representation in
complement to two is had if x > 0
altrimenti 
if x > 0.
11) Shape of standardized analogic wave:
12) Champion expressed in terms of the quantization error:
where e(n) it is the quantization error that in the case of the
rounding is worth 
being D the interval of quantization that is 2- b .
13) Hypothesis to the base of the statistical model in the quantization of the error:
to) the sequence of the error champions sequence is one champion of a stationary accidental process
b) the sequence error is scorrelata with the sequence of the exact champions
c) the error is a noise white man
d) the density of probability of the process error is uniform in the field of values of the quantization error
14) Average, variance and autocorrelationship of the noise of quantization in the rounding case:
15) Average, variance and autocorrelationship of the noise of quantization in the case of troncamento in complement to two:
16) segnale/rumore Relationship:
It is had that in the case of the rounding the segnale/rumore relationship increases of 6dB for every bit that joins to the length of the registry. If it marks them of income exceeds the range of the quantizzatore, it is necessary to reduce the amplitude but that determines also one reduction of the segnale/rumore relationship.
17) Effect of the ended length of the registries in the realization of the numerical filters:
The introduction of not linear elements in some coppers of the structure of the filter is had.
18) Behavior of cycle limit for income 0:
If to a realized numerical filter with ended registries it is applied marks them null for n >n0 is had that the escape grows fainter until to a field of values of not null amplitude and then introduces an oscillating behavior.
19) Band dead woman for a filter of the first order:
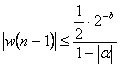
when the income is 0 and the variable one of node |w(n-1)| enters in the band dead woman establishes of the cycles limit.
20) Oscillations from saturation:
The saturation inserts a remarkable error in escape, and in some cases the escape of the filter can begin to oscillate between the maximum limits of amplitude.
21) Hypothesis for the analysis statistics of filters FIR realizes to you in fixed virgola:
to) the error sequence noise sequence is one white man
b) the error sequence has one density of uniform probability on a quantization interval
c) the error sequence e(n) is scorrelata with the income and the escape
such hypotheses are valid in the case mark them of varied income from champion to champion in enough complex way.