Transformed of Fourier

1)    Theorem of Euler on the determination of the coefficients of the series of Fourier :

The coefficients of the series of Fourier are determine to you from the 2 following integral ones :

From the polynomial of Fourier moltiplicando for cosmx (m = 0.1....,n) and integrating between -p and p is had. Analogous multiplying for senmx (m = 0.1....,n) and integrating between -p and p it is had . Having taken advantage of remarkable integral following :

;

;

 

2) Identity of Pitagora Parseval :

If the function f(x) satisfies the condition of Dirichlet and to_n and b_n they are the coefficients of the series of Fourier ž

It is demonstrated taking polynomial trigonometrical, the multiplying it for f(x) and integrating between -p and p it is obtained : having taken advantage of the integrals , e .

 

3) Theorem on the quadratic convergence in average :

To varying of s_n between all polinomi trigonometrical of degree n, the standard deviation it turns out minimal if s_n = s_n where s_n it is the partial sum n-esima of the series of Fourier associated to f.

Squaring it is obtained e replacing

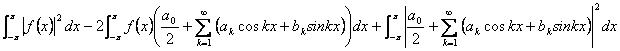
for 1° the term the identity of Parseval can be taken advantage of while for 2° the term the polynomial is taken trigonometrical, it is multiplied for 2f(x) and integral between -p and p it turns out some the thesis.

 

4) Inequality of Bessel :

It is gained from the quadratic convergence in average observing that in how much la function integranda is positive, therefore the result found in that case is valid as inequality.

 

5) Theorem on the punctual convergence :

If f it is a continuous function at times and periodic with period 2p

ž the series of Fourier of the f converges in every point x in which the condition of Dirichlet is satisfied and its sum in such point is worth while if x are one point of continuity for f then the series converges with sum f(x). Being f(x^-) = the value of left limit and f(x ) = the value of the skillful limit.

 

6) Theorem on the uniform convergence :

If f it is a continuous function and with continuous derivative except to more a n° ended than points in which it is however respected the condition n° the 2 of Dirichlet ž series of Fourier of the f converge absolutely and uniform in ".

 

7) Transformed of Fourier associated to f(t) :

where the f(t) it must be absolutely sommabile.

 

8) Antitrasformata di Fourier associated to f(t) :

 

9) Identity of Parseval :

 

10) As to write it marks them f(t) that retort 2N ₀ times marks themf(t):

For means of the convoluzione between (t) marks themf₀ and a train of impulsi