1) Calcola the product to climb _:

(2*1) (3*-1) (1*0) = -1

 

2) Calcola the vectorial product and to say what characterizes:

The vectorial product is a valid operation exclusively in "³ and whose meant it is to find a carrier that he is orthogonal to both the previous carriers, in our case is .

 

3) What is and like varied the direction in the space exchanging the carriers:

Draft of determining of the having matrix the which carriers column the 3 carriers of which to estimate the direction. It is spoken about positive direction in the case that the 3 carriers can are represent to you from medium index and inch of the right hand and negative direction in the case in which they are represents to you from the same fingers but of the left hand.

 

4) That what ¨ a parallelogramma and that what ¨ a parallelepipedo:

A parallelogramma it is a quadrilateral that lies on the having plan sides to two to two parallels. A parallelepipedo it is instead the union of 2 parallelograms situates on 2 plans parallels to you.

 

5) Like varied the volume of a parallelepipedo if to a carrier we replace the sum of a multiple of an other carrier:

It minimally does not change in how much the volume of the parallelepipedo is equal to the determining one of the having matrix as carriers column 3 concurrent chines, are therefore clearly that altering in the way described such matrix, for the rules on the determining one, determining the same one does not vary.

 

5) Like obtaining a carrier of unitary length:

Draft simply to divide it for its norm

 

6) As to estimate the area of a triangle of you concern to us Or, x = , y = :

Draft of a triangle that lies on a plan and therefore its area not of it keeps account della particular position spaces them, the method more express in order to calculate such area is to use the formula where to it is the included angle between the 2 carriers whose value can be calculated through the product to scale .

 

7) How many ways exist in order to characterize a straight one in the space:

1) intersection of 2 plans expressed in cartesian shape

2) Straight passing for a point along the direction characterized from a carrier to it parallel.

How it is passed from the cartesian representation of a straight one in the space to the parametric representation ?

It is gained in terms of span resolving the homogenous system of 2 equations in 3 incognito.

 

8) As it is passed from the parametric representation of a straight one in the space to the cartesian representation :

The equation of the generic point is written, island t for the 3 obtained equations and then 2 to 2 are equaled, the result are the 2 cartesian equations of the plans whose intersection is the straight one.

 

9) When 2 straight ones are sghembe:

When some plan does not exist that contains them both.

 

10) Like currency the distance between 2 straight ones sghembe:

Currency the distance between a point of and a plan to it containing parallel the 2ª straight, gains a cartesian equation of such slowly obtaining a carrier to it normal data from the vectorial product of the carriers directors of the 2 straight ones and in order to find the coefficient d is prevailed that the plan passes for the same point evidenced in the generic equation of the 2ª straight. To such point currency the distance of the point from the plan through the formula

 

11) How many ways exist in order to characterize a plan in the space:

1) Which span of 2 carriers directors and passing for a given point.

2) Plan passing for 3 given points.

3) Plan expressed from one cartesian equation.

 

12) As it is passed from the cartesian representation of a plan to the parametric representation :

The system constituted from an only equation in 3 variable ones is resolved simply and that therefore it will express the solutions which span of 2 carriers directors, it is the parametric shape.

 

13) As it is passed from the parametric representation of a plan to the cartesian representation :

The orthogonal carrier to the plan is written which produced vectorial between the 2 carriers directors, its coordinates correspond to the coefficients of the incognito in the equation of the plan, in order to gain the famous term replace to such equation a point for which the plan must pass.

 

14) As the straight intersection is estimated if two plans are expressed from cartesian equations:

The linear system constituted from the 2 equations of the plans is resolved, being 2 equations in 3 incognito the solutions will be expressed which span of 1 carrier.

 

15) As the straight intersection is estimated if two plans are expressed from parametric equations:

Parametric representation is passed to the correspondent and it is proceeded R-come.sopra.

 

16) As the intersection of two straight ones expressed from parametric equations is estimated:

The generic point of both is written the straight ones equals, finds them therefore the values of the parameters for which the 2 straight ones such values replaced are intersected in respect generic points must give back the same point to you that then is the point of intersection of the 2 straight ones.

 

17) As the intersection of two straight ones expressed from cartesian equations is estimated:

It is passed to the parametric representation and it is proceeded R-come.sopra.

 

18) What is a normal carrier to a plan and qual' it is its I use main:

It is a carrier having like coordinated the coefficients of terms x, y, z of the plan. It is useful in order to determine the straight intersection of 2 plans and in other applications.

 

19) As the intersection between a plan in cartesian shape is found and one straight in parametric shape:

The coordinated generic point of the straight one to the respective ones of the plan is replaced and the value of the parameter in the intersection point is isolated in such a way that replaced in the generic point gives back the same point of intersection.

 

20) Which it is the formula that expresses the distance between a point and a plan:

 

21) As the orthogonal projection of a point on a plan is estimated:

Which intersection between the plan and straight passing for the having point which the carrier is estimated director an orthogonal carrier to the plan.

Conditions of ortogonalità and parallelism between straight plans and

22) When two plans are parallels:

When they have equal or multiple orthogonal carriers.

 

23) When two plans are orthogonal:

When the product to climb of the 2 orthogonal carriers is 0.

 

24) When two straight ones are parallels:

When their carriers directors are equal or multiple and the straight ones pass for 2 various points of the plan.

 

25) When two straight ones are orthogonal:

When the product to scale of the 2 carriers directors is 0.

 

26) When straight and a plan is parallels:

When the product to scale between the carrier director of straight and the orthogonal carrier to the plan is 0.

 

27) When straight and a plan is orthogonal:

When the carrier director of the straight one is equal or multiple of the orthogonal carrier to the plan.

Spins and simmetrie of R³

28) Which matrix is associated to one spin of R³ around to the carrier e1:

 

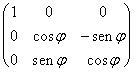
29) As to carry out a spin around to straight whichever of the space:

The straight one must be oriented, and its direction and towards must be indicates you from a payer to it parallel, around to this payer constructs a ortonormale base, according to payer it is found orthogonal to 1° and the third vectorial carrier which produced between the first two carriers. The 3 payers standardize themselves and they gather to form the columns of one orthogonal matrix. The spin around to v like if it were e1, that is is executed with the matrix 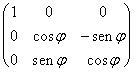dopodichè the result filler in the canonical base through the orthogonal matrix of change of base as soon as constructed.

 

30) Like gaining the formulas of one symmetry respect to an arbitrary plan p :

The orthogonal carrier to the plan is found and its generic value in the equation of the plan is replaced in such a way isolating the value of the parameter t = t_p and observing that in the point to simmetrizzare the parameter it is t = 0 it follows that the parameter in the simmetrizzato point cannot that to be worth 2 * t_p , is replaced this value of the parameter to the generic point of the straight one and ottiengono the equations of the generic simmetrizzato point.

 

31) Like gaining the formulas of the reflection of a point p respect to a point q:

It is observed that the point q is found exactly to half between the point p and its symmetry U_p therefore must be and therefore 2q = p U_p edunque U_p = 2q - p that is the equation of the simmetrizzato point p.